Meet The Teacher

Meet the Teacher - Gemma

On Wednesday afternoon, I was invited into 1/2GA to see how the Year 1 and 2 students were being challenged to think about numbers in a variety of forms. 

Students were initially introduced to the WALT: recognise, model and represent 2, 3 and 4 digit numbers. Gemma expertly broke down into student friendly language with her class – Recognise – look, model – show and represent – make. This allowed students to have an understanding of exactly what Gemma was expecting from them and what they would try to discover in the lesson.

Students who demonstrate that they can read, write make, order, and interpret numbers to 1000 can be described as being able to work with 3-digit numbers. Before achieving this, students have developed fluency in working with 2-digit numbers through activities requiring reading, writing, making, interpreting, and ordering.

Students were hooked into the lesson with a mix of explicit teaching and fun and engaging games, students moved between the two seamlessly, with Gemma taking regular breaks in the games to explain thinking and challenging students to extend their thinking. This started with a fun game of mastermind – where students had to make guesses about which digit might be written in each place value column and then which number this would make.

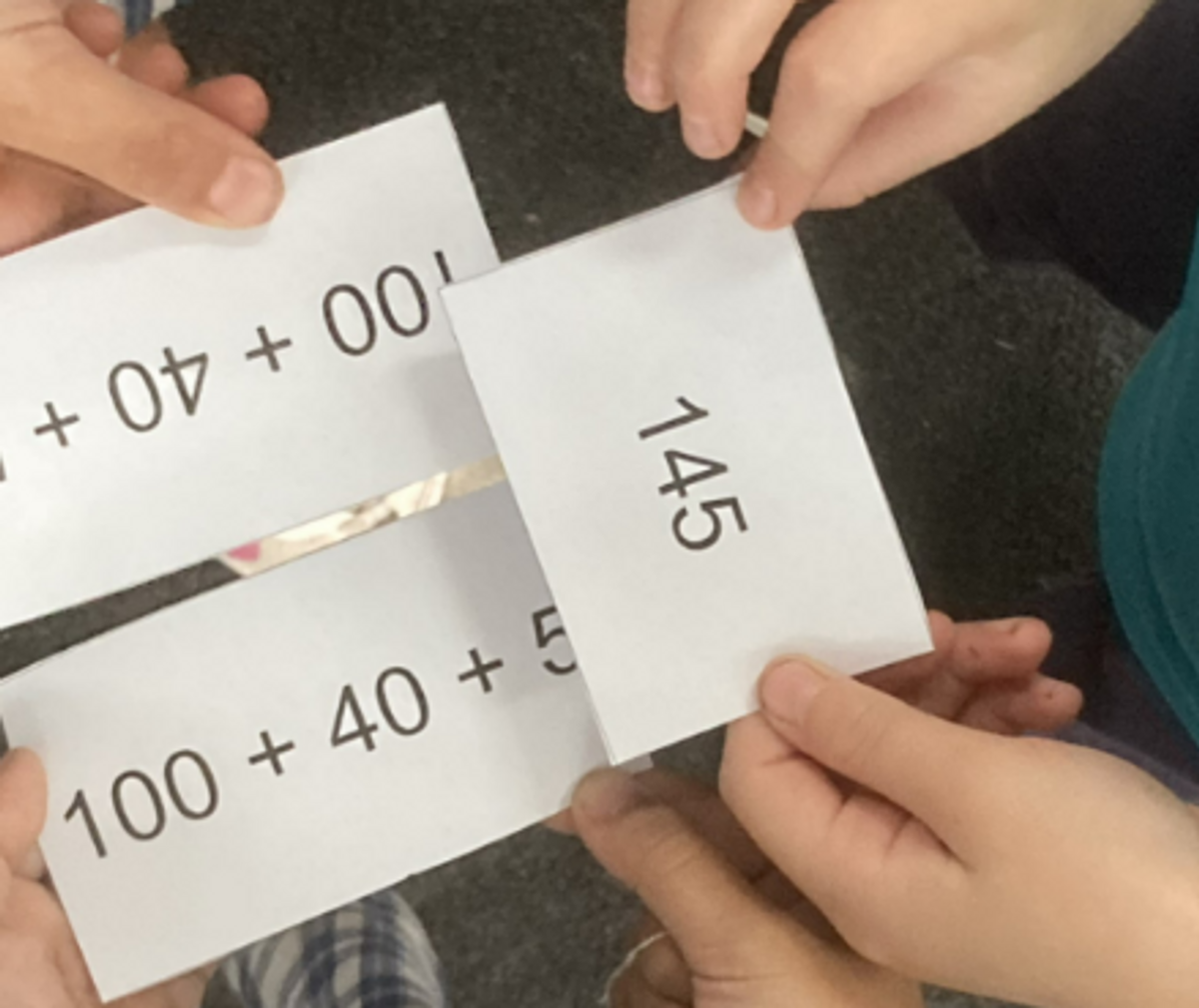
Students were then introduced to the concept of expanded notation; which is writing a number to show the value of each digit. It represents a number as the sum of each of its digits multiplied by their place value (ones, tens, hundreds etc.) For example: 384 = 3 x 10 0 + 8 x 10 + 4 x 1. = 300 + 80 + 4. Students had the opportunity to move around the room looking for a partner, with some students being given a number in expanded form and some being given the number itself, this allowed students to develop their understanding in a fun way, whilst also allowing Gemma to assess which students were showing an understanding of the concept and which might need further support. There was then a chance for Gemma to allow the experts in the room to share their thinking and understanding, thus furthering the understanding of everyone in the room. 

As students moved into the Application part of the lesson, the part of the lesson where students are doing, collaborating, thinking, and applying their mathematical knowledge. Students were presented with two activities for the lesson. An interactive game students could access on the iPad where they could practice their skills in a fun game. Students were able to pick a level that best suited their learning needs. Students were required to match up numbers with their base-10 equivalent as students developed their confidence with one skill, they were allowed to challenge themselves further. 

The second activity allowed students to roll it, make it, and record it using random three digit numbers. Students were required to make a number and show their numbers in a variety of ways. Through exploring 3-digit numbers in this same way, students begin to understand the pattern of groups of three that is the basis of the decimal place value system, ie 1, 1 ten, 1 hundred; 1 thousand, 10 thousand, 100 thousand etc.

To participate in these activities, Gemma used a random partner generator to ensure students can work with different partners and be exposed to different ways of thinking. It was fantastic to see how well students found their partners, with no complaints, and jumped straight into the task. This was a clear sign that the expectations of 12GA were explicit and understood by all students and maximised time on task.

A great teaching strategy on display in Gemma’s room, was when she roamed around the room checking in with all students, and when she spotted great learning and maths conversations on display, she was able to stop the class, share what she had seen and give the student the opportunity to share their thinking with the rest of the class. This reinforced that positive behaviour that had been recognised, whilst also allowing the rest of 12GA to further their level of understanding.

As I moved around the room and watched students in the application section of the lesson it was fantastic to see the principles of the Old Orchard Mathematics Instructional Model on display as students were given the opportunity to consolidate their existing knowledge, collaborate with their peers in a meaningful way, be challenged by both their peers and their teacher and experience a catch – the thing in the lesson that makes it both engaging and relevant to their lives as learners.

I thank Gemma for allowing me to see the wonderful work that is on display in 12GA.

Craig Gooding

Learning Specialist